Absolute value inequalities and equations are a bit tricky to work with. There are basically three cases or situations that can arise when working with them. By guessing and checking we can answer the following three questions.
Tip: We can easily generalize the above result so that we can use this idea as a template when solving equations and inequalities with absolute values in them. ( Assume n > 0 )
Case 1: | X | = n can be solved using X = -n or X = n.
Case 2: | X | < n can be solved using -n < X < n.
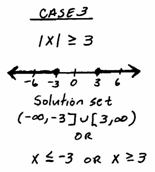
Case 3: | X | > n can be solved using X < -n or X > n.
Use the following steps to solve an absolute value equation or inequality.
Step 1: Isolate the absolute value.
Step 2: Identify the case and apply the appropriate theorem.
Step 3: Solve the resulting equation or inequality.
Step 4: Graph the solution set and express it in interval notation.
Instructional Video: Absolute Value Inequalities
In the three cases listed above notice that we took care to say that n > 0. The next three problems illustrate some of the situations encountered when n = 0. Plug in some numbers and see what happens.
YouTube Videos:








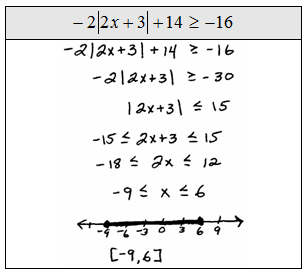
















comment 0 التعليقات:
more_vertsentiment_satisfied Emoticon